

The Mittag-Leffler function of the form E ρ ( z ) = ∑ k = 0 ∞ z k Γ ( k ρ + 1 )was introduced in, where z ∈ C and ρ is an arbitrary positive constant. The Mittag-Leffler function plays a very important role in the solution of fractional-order differential equations. Section snippets Generalized Mittag-Leffler function The conclusion of these studies are presented in Section 4. In Section 3, some application examples of numerical Laplace transform algorithms in fractional calculus are provided. In Section 2 we briefly introduce the Mittag-Leffler function, fractional calculus and numerical inverse Laplace transform algorithms. In this study, Invlap, Gavsteh and NILT algorithms were applied to calculate the inverse Laplace transforms for some simple and complicated fractional-order differential equations. In this paper, we will investigate the validity of numerical inverse Laplace transform algorithms to overcome these difficulties. The rapid growth of fractional-order models leads to the emergence of complicated fractional-order differential equations, and brings forward challenges for solving these complicated fractional-order differential equations. Moreover, some variable-order fractional models and distributed-order fractional models were proposed to understand or describe basic nature in a better way.
A growing number of fractional-order differential equation based models were provided to describe physical phenomena and complex dynamic systems. Nowadays, fractional calculus has been applied extensively in science, engineering, mathematics, and so on, ,. Fractional calculus, developed from the field of pure mathematics, was increasingly studied in various fields, ,. Leibniz raised the possibility of generalizing the operation of differentiation to non-integer orders in 1695. In our study, Invlap, Gavsteh and improved NILT, which is simply called NILT in this paper, are tested using Laplace transform of simple and complicated fractional-order differential equations.įractional calculus is a part of mathematics dealing with derivatives of arbitrary order, ,. However, there is a lack of assessments for applying numerical inverse Laplace transform algorithms in solving fractional-order differential equations. Furthermore, some efforts have been made to evaluate the performances of these numerical inverse Laplace transform algorithms. The quotient-difference algorithm based NILT method is more numerically stable giving the same results in a practical way.
The algorithm was improved using a quotient-difference algorithm in. The NILT method is based on the application of fast Fourier transformation followed by so-called ɛ ‐algorithm to speed up the convergence of infinite complex Fourier series. Gavsteh numerical inversion of Laplace transform algorithm was introduced in, and the NILT fast numerical inversion of Laplace transforms algorithm was provided in.
LAPLACE TRANSFORM CHART SERIES
Based on accelerating the convergence of the Fourier series using the trapezoidal rule, Invlap method for numerical inversion of Laplace transform was proposed in. Direct numerical inversion of Laplace transform algorithm, which is based on the trapezoidal approximation of the Bromwich integral, was introduced in.
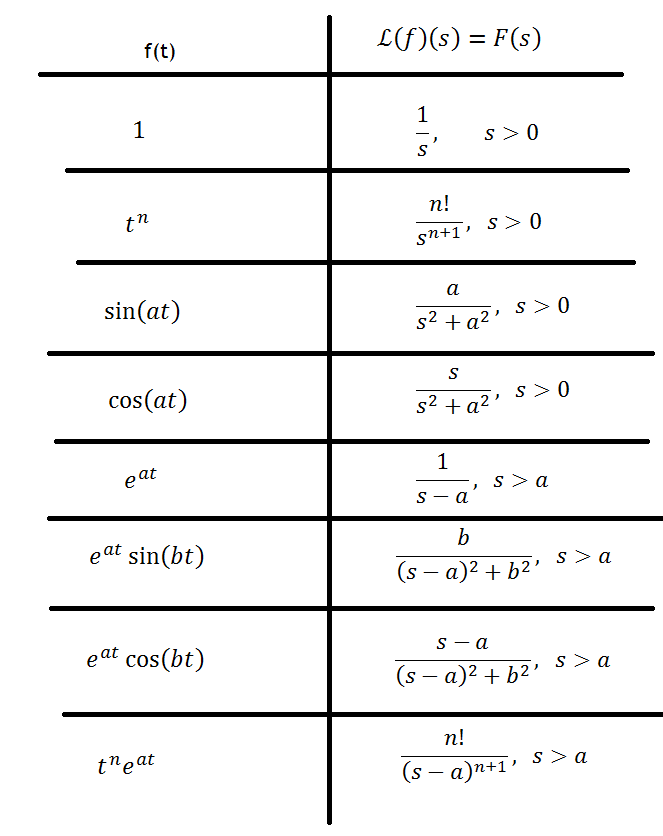
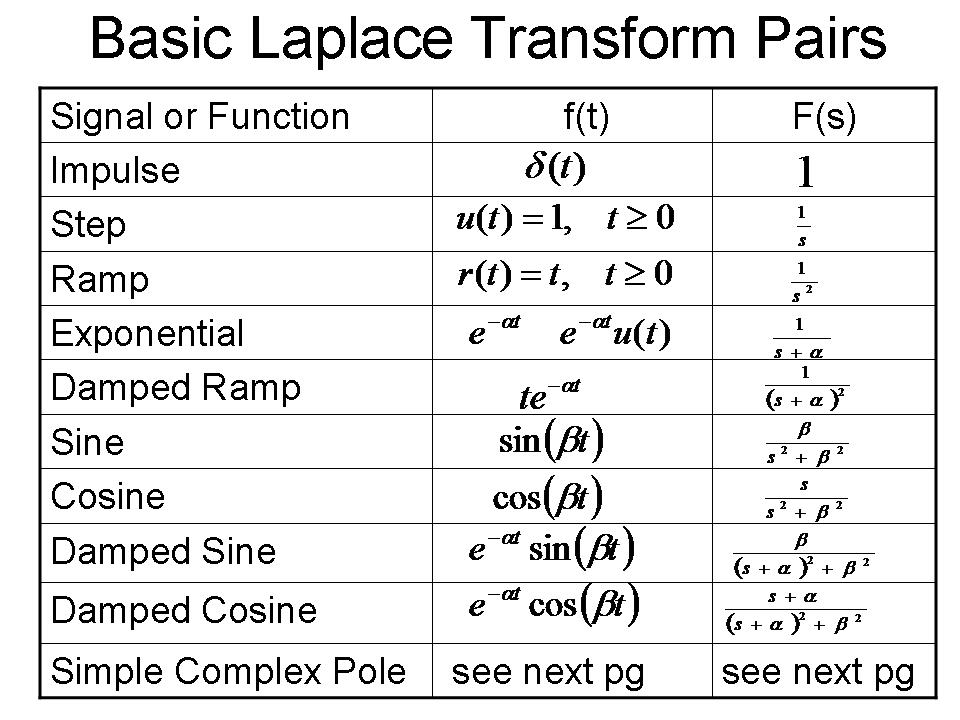
Weeks numerical inversion of Laplace transform algorithm was provided using the Laguerre expansion and bilinear transformations. Many numerical inverse Laplace transform algorithms have been provided to solve the Laplace transform inversion problems. Motivated by taking advantages of numerical inverse Laplace transform algorithms in fractional calculus, we investigate the validity of applying these numerical algorithms in solving fractional-order differential equations. So, the numerical inverse Laplace transform algorithms are often used to calculate the numerical results. For a complicated differential equation, however, it is difficult to analytically calculate the inverse Laplace transformation. The inverse Laplace transformation can be accomplished analytically according to its definition, or by using Laplace transform tables. Inverse Laplace transform is an important but difficult step in the application of Laplace transform technique in solving differential equations. Laplace transform has been considered as a useful tool to solve integer-order or relatively simple fractional-order differential.


 0 kommentar(er)
0 kommentar(er)
